Fundamental Theorem of Calculus Part 1: Integrals and Antiderivatives. As mentioned earlier, the Fundamental Theorem of Calculus is an extremely powerful theorem that establishes the relationship between differentiation and integration, and gives us a way to evaluate definite integrals without using Riemann sums or calculating areas. 5.3 Fundamental Theorem of Calculus 3 Example 2.2. Find the derivative of g(x) = R x 3 p t2 +4dt Example 2.3. Find the derivative of h(u) = R u 3 p y2 +4dy Example 2.4. Find the derivative of f(x) = R ˇ x tan2 tdt Example 2.5. Find the derivative of y= R sin3t 4 e5t2 dt.
- 6.3 1st Fundamental Theorem Of Calculus Ap Calculus Frq
- 6.3 1st Fundamental Theorem Of Calculus Ap Calculus Transcendentals
- 6.3 1st Fundamental Theorem Of Calculus Ap Calculus Multiple Choice
- 6.3 1st Fundamental Theorem Of Calculus Ap Calculus 14th Edition
Following are some of the most frequently used theorems, formulas, and definitions that you encounter in a calculus class for a single variable. The list isn’t comprehensive, but it should cover the items you’ll use most often.
Limit Definition of a Derivative

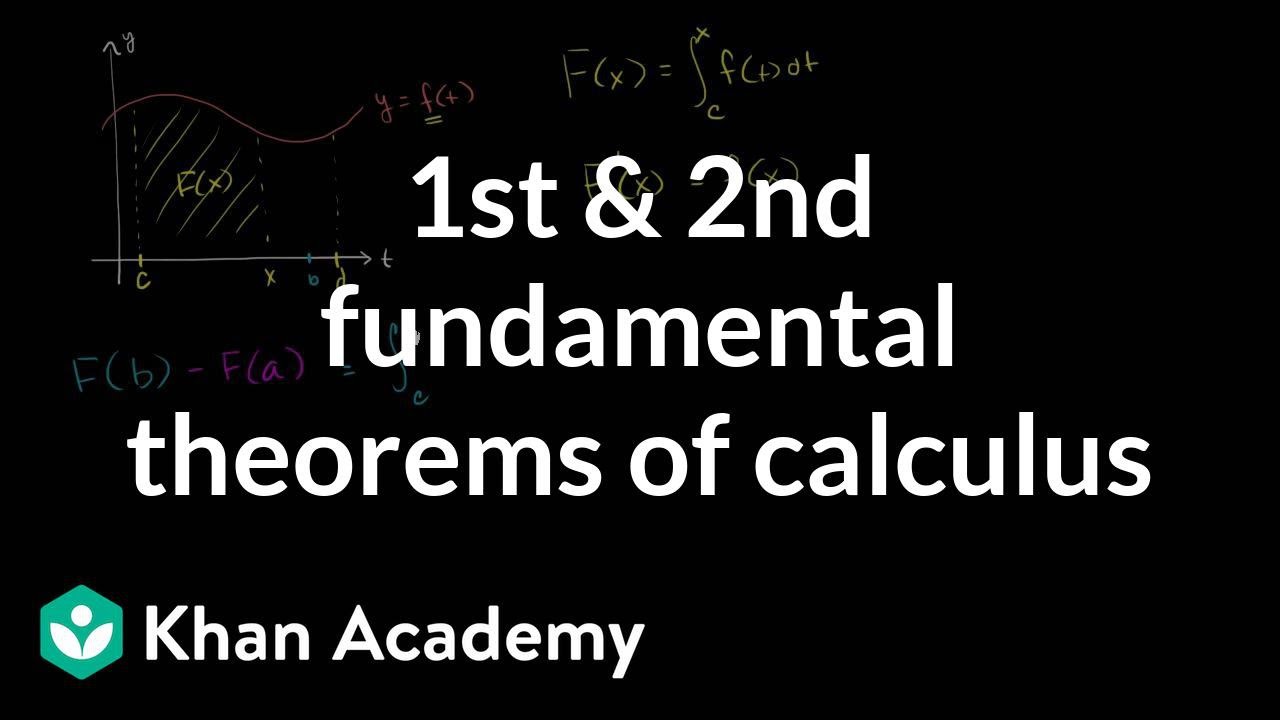
The first part of the theorem, sometimes called the first fundamental theorem of calculus, states that one of the antiderivatives (also called indefinite integral), say F, of some function f may be obtained as the integral of f with a variable bound of integration. This implies the existence of antiderivatives for continuous functions. Fundamental Theorem of Calculus: If f is a continuous function defined on a closed interval a, b and F is an antiderivative of f, then. Steps to use to complete the Fundamental Theorem of Calculus. 1st Integrate the given function (find F(x)). 2nd Find F(b) and F(a) and subtract those values. Examples, from page 431.
Shed the societal and cultural narratives holding you back and let step-by-step Stewart Calculus textbook solutions reorient your old paradigms. NOW is the time to make today the first day of the rest of your life.
Definition: Continuous at a number a
The Intermediate Value Theorem
Definition of a Critical Number
A critical number of a function f is a number c in the domain of f such that either f‘(c)= 0 or f‘(c) does not exist.
Rolle’s Theorem
Let f be a function that satisfies the following three hypotheses:
f is continuous on the closed interval [a, b].
f is differentiable on the open interval (a, b).
f(a)= f(b).
Then there is a number c in (a, b) such that f‘(c)= 0.
The Mean Value Theorem

6.3 1st Fundamental Theorem Of Calculus Ap Calculus Frq
Let f be a function that satisfies the following hypotheses:
f is continuous on the closed interval [a, b].
f is differentiable on the open interval (a, b).
Newton’s Method Approximation Formula
Newton’s method is a technique that tries to find a root of an equation. To begin, you try to pick a number that’s “close” to the value of a root and call this value x1. Picking x1 may involve some trial and error; if you’re dealing with a continuous function on some interval (or possibly the entire real line), the intermediate value theorem may narrow down the interval under consideration. After picking x1, you use the recursive formula given here to find successive approximations:
A word of caution: Always verify that your final approximation is correct (or close to the value of the root). Newton’s method can fail in some instances, based on the value picked for x1. Any calculus text that covers Newton’s method should point out these shortcomings.
The Fundamental Theorem of Calculus
Suppose f is continuous on [a, b]. Then the following statements are true:
The Trapezoid Rule
where
Simpson’s Rule
where n is even and
Following are some of the most frequently used theorems, formulas, and definitions that you encounter in a calculus class for a single variable. The list isn’t comprehensive, but it should cover the items you’ll use most often.
Limit Definition of a Derivative
Definition: Continuous at a number a
The Intermediate Value Theorem
Definition of a Critical Number
A critical number of a function f is a number c in the domain of f such that either f‘(c)= 0 or f‘(c) does not exist.
Rolle’s Theorem
Let f be a function that satisfies the following three hypotheses:
f is continuous on the closed interval [a, b].
f is differentiable on the open interval (a, b).
f(a)= f(b).
Then there is a number c in (a, b) such that f‘(c)= 0.
The Mean Value Theorem
Let f be a function that satisfies the following hypotheses:
f is continuous on the closed interval [a, b].
f is differentiable on the open interval (a, b).
Newton’s Method Approximation Formula
Newton’s method is a technique that tries to find a root of an equation. To begin, you try to pick a number that’s “close” to the value of a root and call this value x1. Picking x1 may involve some trial and error; if you’re dealing with a continuous function on some interval (or possibly the entire real line), the intermediate value theorem may narrow down the interval under consideration. After picking x1, you use the recursive formula given here to find successive approximations:
A word of caution: Always verify that your final approximation is correct (or close to the value of the root). Newton’s method can fail in some instances, based on the value picked for x1. Any calculus text that covers Newton’s method should point out these shortcomings.
The Fundamental Theorem of Calculus
Suppose f is continuous on [a, b]. Then the following statements are true:
6.3 1st Fundamental Theorem Of Calculus Ap Calculus Transcendentals
The Trapezoid Rule
where
6.3 1st Fundamental Theorem Of Calculus Ap Calculus Multiple Choice
Simpson’s Rule
6.3 1st Fundamental Theorem Of Calculus Ap Calculus 14th Edition
where n is even and
